- Location
- the details
Everything.
There are things I know that you never can, and vice-versa.
Everything.
Let me ask you a question?... you're not making any sense at all to me here. How does any of your last few posts tie into the topic of this thread?
Nonsense. Analogous objects might exist under different physical laws, but they wouldn't be black holes. The geometry of causality in our universe is what makes them possible in the first place.Let me ask you a question?
"Is it possible to prove black holes exist without using Relativity?".
I know that the answer to that question is "yes".
For every object in the universe, using just classical mechanics, there is a circular orbit radius where the orbital velocity equals the speed of light.Nonsense. Analogous objects might exist under different physical laws, but they wouldn't be black holes. The geometry of causality in our universe is what makes them possible in the first place.
In a Galilean universe, the only speed of light which makes the equations of electromagnetics work is infinity. So, not really. That value turns out not to work for other reasons, but all that means is you can't have electromagnetics without relativity. Without electromagnetics you can't have humans.For every object in the universe, using just classical mechanics, there is a circular orbit radius where the orbital velocity equals the speed of light.
I must wonder, have you ever tried to solve a system where you had more variables than equations?In a Galilean universe, the only speed of light which makes the equations of electromagnetics work is infinity. So, not really. That value turns out not to work for other reasons, but all that means is you can't have electromagnetics without relativity. Without electromagnetics you can't have humans.
But let's ignore that. In a Galilean universe there is no upper speed -- relativity is precisely what you get when you require the universe to have a speed limit. Hence, even if your photon-analogues have mass and therefore can travel at finite (and varying!) speed, that's got no bearing on whether or not you can climb out of a black hole. If there is no upper speed then you can, and therefore it isn't black.
There's a third solution, where all four dimensions are space-like. In that one you can reach infinite speed using finite energy, so it doesn't have black holes either.
I must wonder, have you ever tried to solve a system where you had more variables than equations?
*shrugs*
*shrugs*
Maybe nothing, maybe everything.
FTL is weird like that.
It is an answer. It's just not the one you wanted.
Perhaps I should step away from this thread. Which I will do from here on out.... are you interested, at all, in having a conversation here? Because, look, dropping elementary math truisms off in here -- whose relevance to the actual physical concepts involved in FTL is non-existent -- as if they were deep insights got old real fast, and isn't advancing us anywhere.
It sounds like you make the frame of reference of the ship before the jump privileged here. That contradicts the fundamental assertion of relativity that all frames of reference that can be constructed through Lorentz transformations are equally valid.More seriously, a while back I played around with a Lorentz transformation to figure out how a system analogous to a Warp Drive would behave based on the velocity of the ship prior to going to warp. I came to the following conclusions:
1: If the ship is (roughly) stationary relative to the destination prior to going to warp, it behaves like most space opera. Roughly speaking the observed travel time aboard the ship will match the observed travel time at the destination.
2: If the ship is moving towards the destination prior to warp, the observed travel time aboard will be decreased, but the observed travel time at the destination will be increased.
3: If the ship is moving away from the destination prior to going to warp, observed travel time aboard the ship will increase, but observed travel time at the destination will decrease. If your realspace velocity away from the destination is greater than the inverse of your warp 'velocity', then the observed travel time at your destination will actually be negative and you'll probably wind up in a branch timeline.
Enh, that isn't quite true. There is a symmetry-breaking privileged frame here -- it's whatever frame the warp drive "starts up" in.It sounds like you make the frame of reference of the ship before the jump privileged here. That contradicts the fundamental assertion of relativity that all frames of reference that can be constructed through Lorentz transformations are equally valid.
We already know from Quantum Mechanics(well, classical probability too) that information can technically be instantaneous - that is to say, we can know about the quantum state of some object at event (t,x) before our own clock says it's t+x. Which is a no brainer; even classically, if I have a pair of shoes, put both shoes into different boxes(without me knowing which one is in which), send one of the boxes to Mars, and then open the box left on Earth to observe that it's a left shoe, then I'll instantaneously know that the shoe on mars is a right shoe, even though Mars is 3 light minutes away - ie. I instantaneously acquire information about an object 3 light minutes away. Information is ultimately something in our minds, not something that materially exists.Has anyone ever considered that the "speed of light" is a really misleading name? I mean, yes that is the speed at which light travels, but its getting cause and effect backwards. Light travels at the speed that it does because that is the maximum speed that anything can travel.
I think we should call it the "speed of information"
You don't need any fancy math to justify why there should be a maximum speed at which information can propagate. There can only be four possible cases:
- Information has no speed and does not propagate. There is only void
- Information has no speed, all information is available everywhere. Nothing could exist because everything would be affected by an infinite amount of other stuff.
- Information has variable speed. The information about the rate of change in information speed is itself information.
- Information has some fixed speed.
To counterpoint: The speed of light is what it is because that's the apparent speed of a null geodesic -- a zero-length four-dimensional path -- as seen from a three-dimensional perspective.Has anyone ever considered that the "speed of light" is a really misleading name? I mean, yes that is the speed at which light travels, but its getting cause and effect backwards. Light travels at the speed that it does because that is the maximum speed that anything can travel.
I think we should call it the "speed of information"
You don't need any fancy math to justify why there should be a maximum speed at which information can propagate. There can only be four possible cases:
- Information has no speed and does not propagate. There is only void
- Information has no speed, all information is available everywhere. Nothing could exist because everything would be affected by an infinite amount of other stuff.
- Information has variable speed. The information about the rate of change in information speed is itself information.
- Information has some fixed speed.
No that's not correct as far as I can tell. If by "angle" you man the euclidean angle between linear worldlines those are not Lorentz-invariant by definition. What is invariant is the dt^2-|dx|^2 which is positive for time-like separations, zero for light-like and negative for space-like. Those cathegories stay fixed between Lorentz trafos but within them anything goes. You define a kind of pseudo-angle that does stay constant I think but that's kind of pointless.Enh, that isn't quite true. There is a symmetry-breaking privileged frame here -- it's whatever frame the warp drive "starts up" in.
If you imagine that we have a warp drive that boosts you to 5c... well that's a Lorentz boost greater than infinity so lol, but we'll ignore that for now. Anyway, 5c in which frame? Presumably in the frame where you started the device.
The rest basically follows from there. The Lorentz-invariant truth is that the 'angle' (which is actually ill-defined because FTL but again ignoring that for the moment) between the 'stationary' worldline and the 'warp-speed' worldline is equivalent to 5c of boost, and that relative measurement will be the same in all frames -- but there's no rule that says that any of the other results have to be invariant, and in fact if they were relativity would be much less interesting.
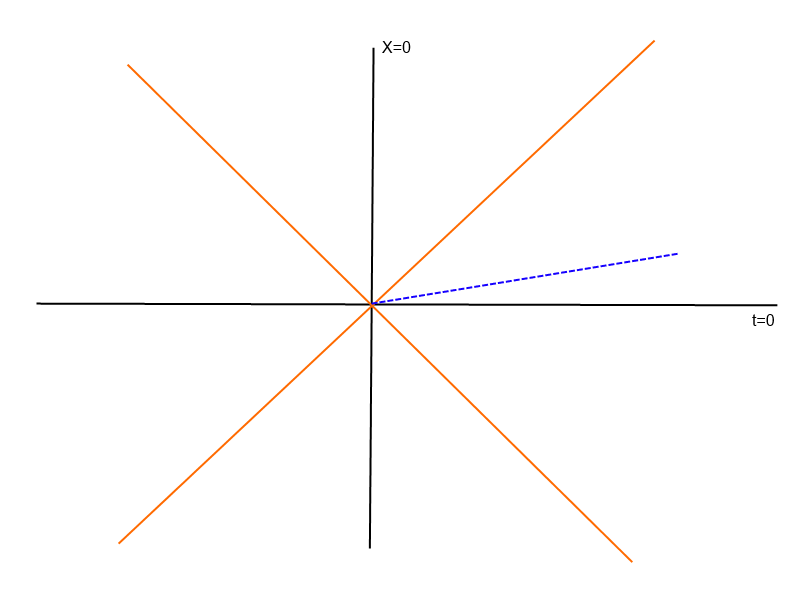
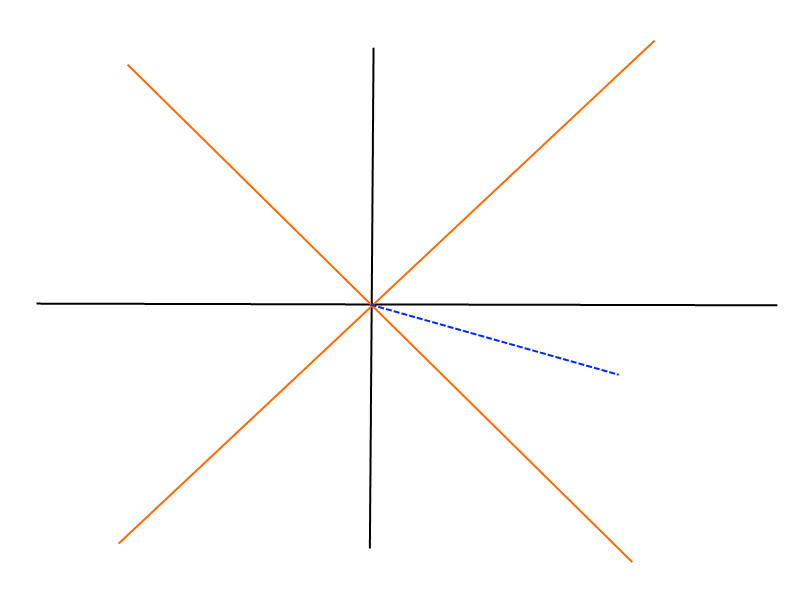
It sounds like you make the frame of reference of the ship before the jump privileged here. That contradicts the fundamental assertion of relativity that all frames of reference that can be constructed through Lorentz transformations are equally valid.
And I see no reason why the first frame of reference should be privileged in any way. The second one is just as valid.
Travel time aboard the ship is the proper time of the ship. Observed travel time of the ship at the destination is also a form of proper time -- depending on the details of the destination frame it will not agree with anyone else's observation of the ship's travel time, but everyone will agree that the destination observed X amount of time while waiting for the ship to arrive.1: If the ship is (roughly) stationary relative to the destination prior to going to warp, it behaves like most space opera. Roughly speaking the observed travel time aboard the ship will match the observed travel time at the destination.
2: If the ship is moving towards the destination prior to warp, the observed travel time aboard will be decreased, but the observed travel time at the destination will be increased.
3: If the ship is moving away from the destination prior to going to warp, observed travel time aboard the ship will increase, but observed travel time at the destination will decrease. If your realspace velocity away from the destination is greater than the inverse of your warp 'velocity', then the observed travel time at your destination will actually be negative and you'll probably wind up in a branch timeline.
Nope, I mean the hyperbolic angle between worldlines.No that's not correct as far as I can tell. If by "angle" you man the euclidean angle between linear worldlines those are not Lorentz-invariant by definition. What is invariant is the dt^2-|dx|^2 which is positive for time-like separations, zero for light-like and negative for space-like. Those cathegories stay fixed between Lorentz trafos but within them anything goes. You define a kind of pseudo-angle that does stay constant I think but that's kind of pointless.